
In his 1938 paper, Lévy wrote [see Edgar]
Moreover, it has the very curious property that, if [the dragon curve] were materialized, one could tile the plane with it, i.e., one can cover the plane with identical copies of this curve such that no two copies intersect, and such that no space is left uncovered.
Here is one way to visualize why this tiling works. The plane can certainly by tiled by unit squares. Divide each unit square into four congruent isosceles right triangles, each a congruent copy of L0, the isosceles right triangle with base along the unit interval and vertex at (1/2, 1/2). This was the triangle used in the description of of the construction of the Levy dragon. Applying the first step of the Lévy dragon construction to each of these triangles produces four congruent copies of L1 that still cover the unit square (see Figure 1).
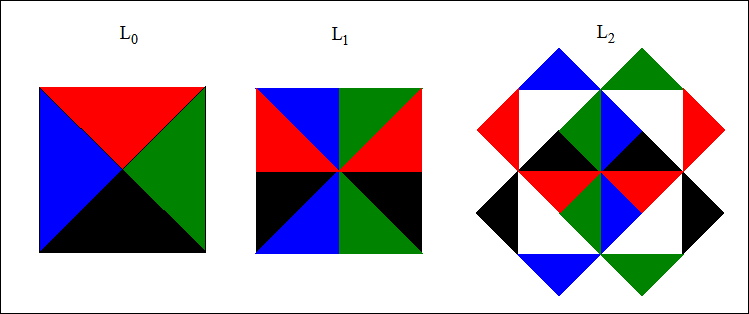
Figure 1
Now apply the next step of the construction to each of these regions to get four congruent copies of L2. Some of the new triangles will now point outward, leaving what appears to be empty space in the square in the third diagram in Figure 1. But of course, we are also doing this construction to each of the surrounding unit squares, and some of the triangles from those surrounding squares produce new triangles that fill in the gaps. Try it with the following animation of steps using copies of L2 from the 8 surrounding squares to produce the following tiling.
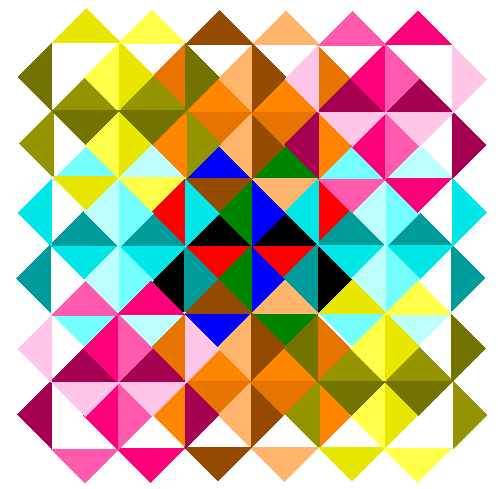
 Notice in the tilings using L2 that the unit square (shown to the right) can be split
into 4 smaller squares of area 1/4 that resemble the original pattern of
four isosceles right triangles in the tiling with L0. We saw how
the plane continues to be tiled in going from L0 to L1 to
L2. Because the tiling for L2 consists of the same pattern
as for L0, only on a smaller scale, the same construction would
show that L3 and L4 continue to tile the plane.
Notice in the tilings using L2 that the unit square (shown to the right) can be split
into 4 smaller squares of area 1/4 that resemble the original pattern of
four isosceles right triangles in the tiling with L0. We saw how
the plane continues to be tiled in going from L0 to L1 to
L2. Because the tiling for L2 consists of the same pattern
as for L0, only on a smaller scale, the same construction would
show that L3 and L4 continue to tile the plane.