IFS Construction Kit Gallery

All these images were created with IFS Construction Kit. Click on an image for a larger view. Click on the corresponding ifs file to view the functions systems, or download the file so you can open it in IFS Construction Kit.

Sierpinski converging to his triangle

Durer's Pentagons

Barnsley's Spleenworth Fern

Examples from Gerald Edgar, Measure, Topology, and Fractal Geometry, Springer-Verlag, 1990. [ifs file]

Heighway Dragon	Twin Dragon	Levy Dragon	McWorter's Pentigree Dragon
Pentadentrite	Eisenstein Fractions	Barnsley's Wreath	Koch Snowflake

Examples from Kevin Lee and Yosef Cohen, Fractal Attraction: A Fractal Design System for the Macintosh, Academic Press, 1991. [ifs file]

Eiffel Tower	Nautilus	Spiral 1	Spiral 3
Square Snowflake	Starfish

Examples of tilings from "Fractal Tilings in the Plane," Richard Darst, Judith Palagallo, and Thomas Price, Mathematics Magazine, Vol. 71, No. 1, February 1998, 12-23. [ifs file]

Horizontal Tiling	Terdragon Tiling	Gosper Snowflake Tiling	4-rep Tile
4-rep Tile with Symmetry	5-rep Tile with Symmetry

Examples from "Number Systems With a Complex Base: A Fractal Tool for Teaching Topology," Daniel Goffinet, American Mathematical Monthly, Vol. 98, No. 3 (March 1991), 249-255. [ifs file]

b = −0.62e^2π/7	b = −0.697e^2π/5	b = 0.2+0.6i	b = 0.5 + 0.5i
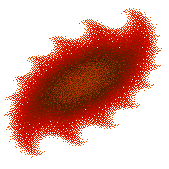b = 0.8 + 0.2i	b = 0.65 − 0.3i

Examples of Triangle Fractals motivated by Fractal World by Dave Ryan (website no longer available) [ifs file]

Sierpinski Triangle	$3 row triangle fractal$ 3 row triangle Triangle	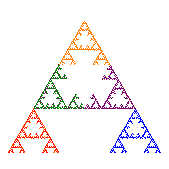3 row fractal with rotations	$4 row triangle fractal$ 4 row triangle fractal
2 rows with reflective symmetry	Opposite reflective symmetry

Examples from Paul Bourke's website on fractals and chaos [ifs file]

Chaos	Leaf	Maple Leaf	Spiral
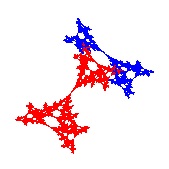Mandelbrot like	Tree	Tree

Examples of color stealing

Input Image	Heighway Dragon	Input Image	Snowflake
Input Image	Fern	Input Image	Spiral
Input Image	Koch Snowflake

Examples of fractal ferns from Ferns of the Canberra Region, a website maintained by David Nicholls and Christopher Nicholls. [ifs file]

Barnsley's Fern (modified pinnae)

Culcita (=Calochlaenia) dubia Fern

"Fishbone" Fern

Cyclosorus Fern

Examples of Pythagorean Trees

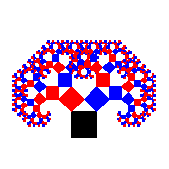45 degrees

60 degrees

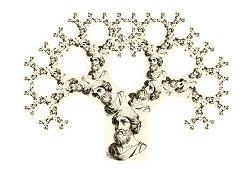Pythagorus as a 45 degree tree!

The tips of a 10 degree tree

Examples of Koch Curves with n-gons [ifs file]

(4,1/3)-Koch Curve

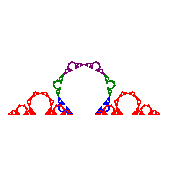(7,0.15)-Koch Curve

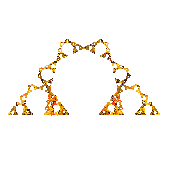(7,0.20)-Koch Curve

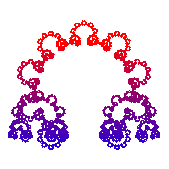(10,1/5)-Koch Curve

Examples of Fractal Movies. Click on an image to start the movie. All of these were constructed using the Fractal Movie Creator in IFS Construction Kit.

Heighway Dragon to Sierpinski Triangle	Dancing Heighway Dragon	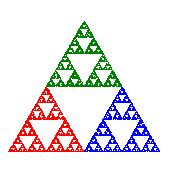Sierpinski Triangle to Fern	Dancing Sierpinski
Rotating Starfish

Examples of Symmetric Fractals using a single affine transformation or a given IFS, then modified using a cyclic or dihedral symmetry group. For an explanation of how these were created, see the Help section. [IFS file]

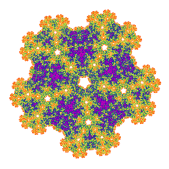Z₅ Symmetry

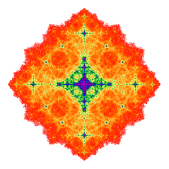Koch curve using D₄ symmetry group

Stained Glass Window (with Z₆ Symmetry)

Heighway Dragon using Z₂ symmetry group

The following examples are from Symmetry in Chaos: A Search for Pattern in Mathematics, Art and Nature byMichael Field and Martin Golubitsky, Oxford University Press (Edition 1, 1992/95) and SIAM (Edition 2, 2009) [IFS file]

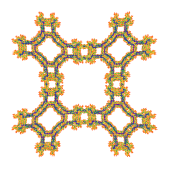
Astigmatism (D₄)	Cashmire (Z₅₀)	Catherine Wheel (Z₉)	Doily (D₈)
Fifty Nations (Z₅₀)	Sierpinski Pentagon (Z₅)	Snowflake (D₆)