
|
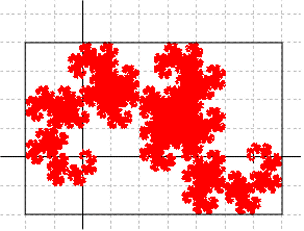
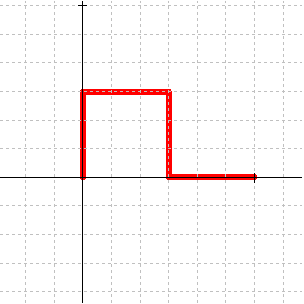
Start the construction of the Heighway dragon with a segment of length 1 along the x-axis. Two iterations produces the figure to the left that has a vertical segment along the y-axis. The left edge is at x = 0, the top edge at y = 0.5, the right edge at x = 1, and the bottom edge at y = 0. The grid size is 1/6 x 1/6. |
|
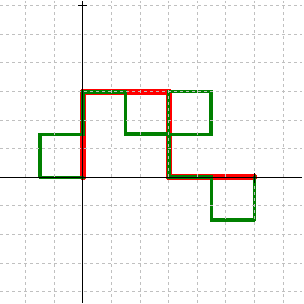
Each iteration produces either horizontal/vertical segments (even iteration) or segments at 45°/135° (odd iteration). The odd iterations will expand the size outward. The next even iteration will keep the size but make the segments vertical or horizontal. Here is the result after the 4th iteration. The left edge is extended by (1/2)2 = 1/4. The top and right edges remain the same. The bottom edge is also extended by 1/4. |
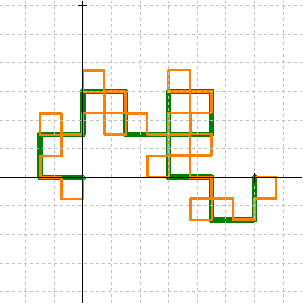
|
At iteration 6, the left and bottom edges remain the same but the top and right edges have now been extended by (1/2)3 = 1/8 |
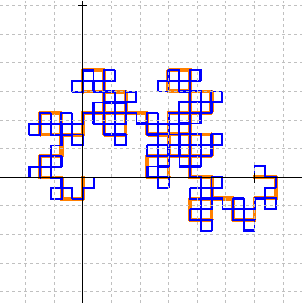
|
At iteration 8, the left and bottom edges are extended another (1/2)4 = 1/16 while the top and right remain the same. |
This pattern continues with the extension of the left/bottom edges alternating with the extension of the top/right edges. The growth in the size of the Heighway dragon is therefore given by the following geometric series
| Left | \[{\left( {\frac{1}{2}} \right)^2} + {\left( {\frac{1}{2}} \right)^4} + {\left( {\frac{1}{2}} \right)^6} + \ldots = \frac{{\frac{1}{4}}}{{1 - \frac{1}{4}}} = \frac{{\frac{1}{4}}}{{\frac{3}{4}}} = \frac{1}{3}\] |
| Top | \[\left( {\frac{1}{2}} \right) + {\left( {\frac{1}{2}} \right)^3} + {\left( {\frac{1}{2}} \right)^5} + \ldots = \frac{{\frac{1}{2}}}{{1 - \frac{1}{4}}} = \frac{{\frac{1}{2}}}{{\frac{3}{4}}} = \frac{2}{3}\] |
| Right | \[{\left( {\frac{1}{2}} \right)^3} + {\left( {\frac{1}{2}} \right)^5} + {\left( {\frac{1}{2}} \right)^7} + \ldots = \frac{{\frac{1}{8}}}{{1 - \frac{1}{4}}} = \frac{{\frac{1}{8}}}{{\frac{3}{4}}} = \frac{1}{6}\] |
| Bottom | \[{\left( {\frac{1}{2}} \right)^2} + {\left( {\frac{1}{2}} \right)^4} + {\left( {\frac{1}{2}} \right)^6} + \ldots = \frac{{\frac{1}{4}}}{{1 - \frac{1}{4}}} = \frac{{\frac{1}{4}}}{{\frac{3}{4}}} = \frac{1}{3}\] |