Pentadentrite

The pentadentrite is a variation of the McWorter's pentigree. Starting from the origin, draw a line segment of length r at an angle A. Turn left by 72° and draw another line segment of length r. Next turn right by 72° and draw a third line segment of length r. The remaining three line segments, each of length r, are formed by making a right turn of 144° followed by two left turns of 72°. The end of the last line segment will be at the point (1,0).

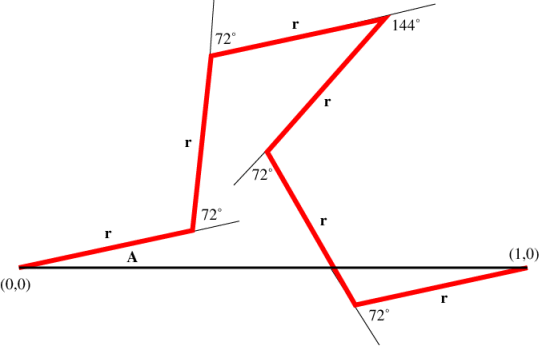
The six red line segments in the figure give the first iteration for this construction. The scaling factor r and the angle A must be chosen so that the endpoint of the last segment is at the point (1,0).

The following calculations show that \(r = \sqrt {\frac{{6 - \sqrt 5 }}{{31}}} = 0.34845\) and A = 11.81858573°.

• Calculation using geometry and trigonometry
• Calculation using complex numbers and vectors

It is now possible to determine how each of the six rotated segments must be translated. For example, the first segment must be scaled by r and rotated through the angle A. The second segment is scaled by r, rotated through an angle of A + 72°, then translated to (r sin A, r sin A). Similar calculations with the other segments led to the following IFS [Details]:
 

\({f_1}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} {0.341} & { - 0.071} \\ {0.071} & {0.341} \\ \end{array}} \right]{\bf{x}}\)	scale by r, rotate by A = 11.819°
\({f_2}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} { 0.038} & { - 0.346} \\ {0.346} & { 0.038} \\ \end{array}} \right]{\bf{x}} + \left[ {\begin{array}{*{20}{c}} {0.341} \\ {0.071} \\ \end{array}} \right]\)	scale by r, rotate by A+72° = 83.819°
\({f_3}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} {0.341} & {-0.071} \\ { 0.071} & {0.341} \\ \end{array}} \right]{\bf{x}} + \left[ {\begin{array}{*{20}{c}} {0.379} \\ {0.418} \\ \end{array}} \right]\)	scale by r, rotate by A = 11.819°
\({f_4}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} { - 0.234} & {0.258} \\ { - 0.258} & { - 0.234} \\ \end{array}} \right]{\bf{x}} + \left[ {\begin{array}{*{20}{c}} {0.720} \\ {0.489} \\ \end{array}} \right]\)	scale by r, rotate by A−144° = −132.181°
\({f_5}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} {0.173} & {0.302} \\ { - 0.302} & {0.173} \\ \end{array}} \right]{\bf{x}} + \left[ {\begin{array}{*{20}{c}} {0.486} \\ {0.231} \\ \end{array}} \right]\)	scale by r, rotate by A−72° = −60.181
\({f_6}({\bf{x}}) = \left[ {\begin{array}{*{20}{c}} {0.341} & { - 0.071} \\ {0.071} & {0.341} \\ \end{array}} \right]{\bf{x}} + \left[ {\begin{array}{*{20}{c}} {0.659} \\ { - 0.071} \\ \end{array}} \right]\)	scale by r, rotate by A = 11.819°

[Enlarge]

Angle 72
Axiom F
F —> F+F−F−−F+F+F
(this will be rotated from the IFS constructed curve)

First three iterations of the L-system

Four iterations

The pentadentrite is self-similar with 6 non-overlapping copies of itself, each scaled by the factor r < 1. Therefore the similarity dimension, d, of the attractor of the IFS is the solution to

\[\sum\limits_{k = 1}^6 {{r^d}} = 1 \quad \Rightarrow \quad d = \frac{{\log (1/6)}}{{\log (r )}} = 1.6995\]

This is called a pentadentrite because five copies of the limit set fit perfectly together to form the following shape [Details]:

[Enlarge]

 

1. Edgar, Gerald A. Measure, Topology, and Fractal Geometry, Springer-Verlag, 1990.